Non-Periodic Phenomena in Variable Stars
IAU Colloquium, Budapest, 1968
PHOTOMETRIC RESEARCH ON RS CVn AT THE CATANIA
ASTROPHYSICAL OBSERVATORY
S. CATALANO and M. RODONO
Catania Astrophysical Observatory, Italy
SUMMARY
On the ground of extensive photoelectric observations of RS CVn made at
Catania since 1963 several photometric peculiarities of this system are
analysed.
A good deal of hypotheses have been proposed to explain the
peculiarities of the system RS CVn (e.g. Sitterly 1930; Mergentaler
1950; Catalano and Rodono 1967). However, as the accumulation of
photometric and spectroscopic observations has been increasing, none of
these hypotheses seems to be completely correct.
Since 1963 photoelectric observations were collected at Catania in order to
reach a description as complete as possible for the properties of this system.
We should like to review here these properties as far as concerns:
1. light curve variation
2. colour index outside eclipses
3. variation of the primary minimum depth
4. orbital period variation and displacement of the secondary minimum
5. spectral peculiarities.
LIGHT CURVE VARIATION
The light curve of RS CVn shows fairly regular variations, in contrary
to many systems having irregular fluctuations or humps at certain phases.
The luminosity of the system outside eclipses is perturbed by a wave-like
distortion (Fig. 1). This distortion, maintaining its shape, moves in
the sense of decreasing phases. In the Fourier expansion of rectified
light curve, all coefficients are negligible compared to those of the
cos phi and sin phi terms (Chisari and Lacona, 1965).
Therefore the observed light curve outside eclipses may be well represented
by the following simple equation:
L[phi, Theta(t)] = L_0 - Delta L cos [phi - Theta(t)]
where phi is the phase angle, and Theta, variable in time, gives the phase
angle of the minimum of the wave-like distortion relatively to the primary
minimum.
The Theta mean values for each year were derived from the Catania
observations by the method of least-squares using the above-mentioned
equation. Three values were also derived from Keller and Limber's (1951)
and Popper's (1961) observations, but they are somewhat uncertain because
their light curves are not complete.
 Fig. 1. Observed light curves.
Fig. 1. Observed light curves.
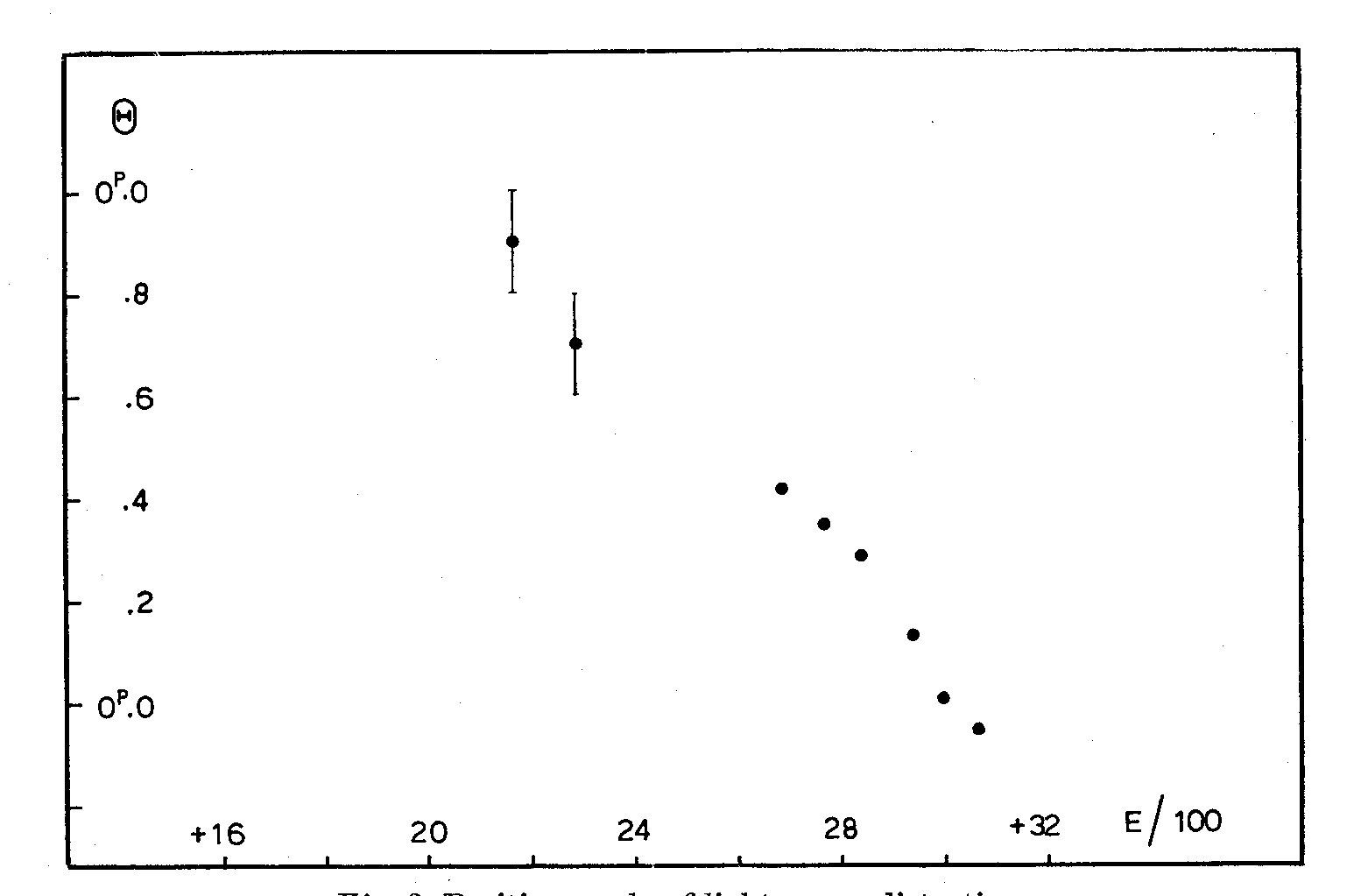 Fig. 2. Position angle of light curve distortion.
Fig. 2 shows the Theta values versus the number of orbital period from an
assumed initial epoch (JD 2425249.028; Schneller, 1928). Because of the
uncertainty of the first three values of Theta it is difficult to determine
the exact period in which Theta reaches the same value. On the hypotheses
that Keller and Limber's observations and ours up to 1966 belong to the
same cycle, we made a rough estimate of 2400 orbital periods (Catalano
and Rodono 1967). Using new observations obtained during 1967 and 1968,
it seems that Keller and Limber's observations belong to a preceding
cycle. Therefore a more suitable value might be about 800 orbital
periods. Limiting ourselves to the Catania observations this period
would be even smaller.
COLOUR INDEX OUTSIDE ECLIPSES
The amplitude of the distortion is larger at longer wavelengths. The
data in the following Table refer to the observations of 1968:
U B V
0.10m 0.14m 0.17m
Consequently the light outside eclipses is bluer at its minimum than at
its maximum. This appears clearly in Figs. 3a and 3b, where for 1967 and
1968 the mean light curves and the colour index variations Delta(B -V), both
outside eclipses, are reported.
This behaviour of the colour index, as observed in many systems showing
distortions of their light curves, has been discussed by Mergentaler (1950).
He supposed that gaseous streams of negative ions of hydrogen of different
optical thickness could cause the observed distortions in the light curve.
If the distortion of RS CVn is due to this reason, the absorbing matter should
have an equilibrium configuration, because the wave-like distortion, as
we have seen, maintains its shape with time. We had suggested (Catalano
and Rodono 1967) that a ring around the equatorial plane of the primary
component might cause the distortion of the light curve and its shift
with time. This idea was supported primarily by the good agreement
between the previously determined period of the distortion shift (2400 P)
and the theoretical period of precession of the equator, which was
assumed to have inclined to the orbital plane. But the new estimate of
the period of the light variation, as previously reported, is too short
to be compared with the theoretical one.
Fig. 2. Position angle of light curve distortion.
Fig. 2 shows the Theta values versus the number of orbital period from an
assumed initial epoch (JD 2425249.028; Schneller, 1928). Because of the
uncertainty of the first three values of Theta it is difficult to determine
the exact period in which Theta reaches the same value. On the hypotheses
that Keller and Limber's observations and ours up to 1966 belong to the
same cycle, we made a rough estimate of 2400 orbital periods (Catalano
and Rodono 1967). Using new observations obtained during 1967 and 1968,
it seems that Keller and Limber's observations belong to a preceding
cycle. Therefore a more suitable value might be about 800 orbital
periods. Limiting ourselves to the Catania observations this period
would be even smaller.
COLOUR INDEX OUTSIDE ECLIPSES
The amplitude of the distortion is larger at longer wavelengths. The
data in the following Table refer to the observations of 1968:
U B V
0.10m 0.14m 0.17m
Consequently the light outside eclipses is bluer at its minimum than at
its maximum. This appears clearly in Figs. 3a and 3b, where for 1967 and
1968 the mean light curves and the colour index variations Delta(B -V), both
outside eclipses, are reported.
This behaviour of the colour index, as observed in many systems showing
distortions of their light curves, has been discussed by Mergentaler (1950).
He supposed that gaseous streams of negative ions of hydrogen of different
optical thickness could cause the observed distortions in the light curve.
If the distortion of RS CVn is due to this reason, the absorbing matter should
have an equilibrium configuration, because the wave-like distortion, as
we have seen, maintains its shape with time. We had suggested (Catalano
and Rodono 1967) that a ring around the equatorial plane of the primary
component might cause the distortion of the light curve and its shift
with time. This idea was supported primarily by the good agreement
between the previously determined period of the distortion shift (2400 P)
and the theoretical period of precession of the equator, which was
assumed to have inclined to the orbital plane. But the new estimate of
the period of the light variation, as previously reported, is too short
to be compared with the theoretical one.
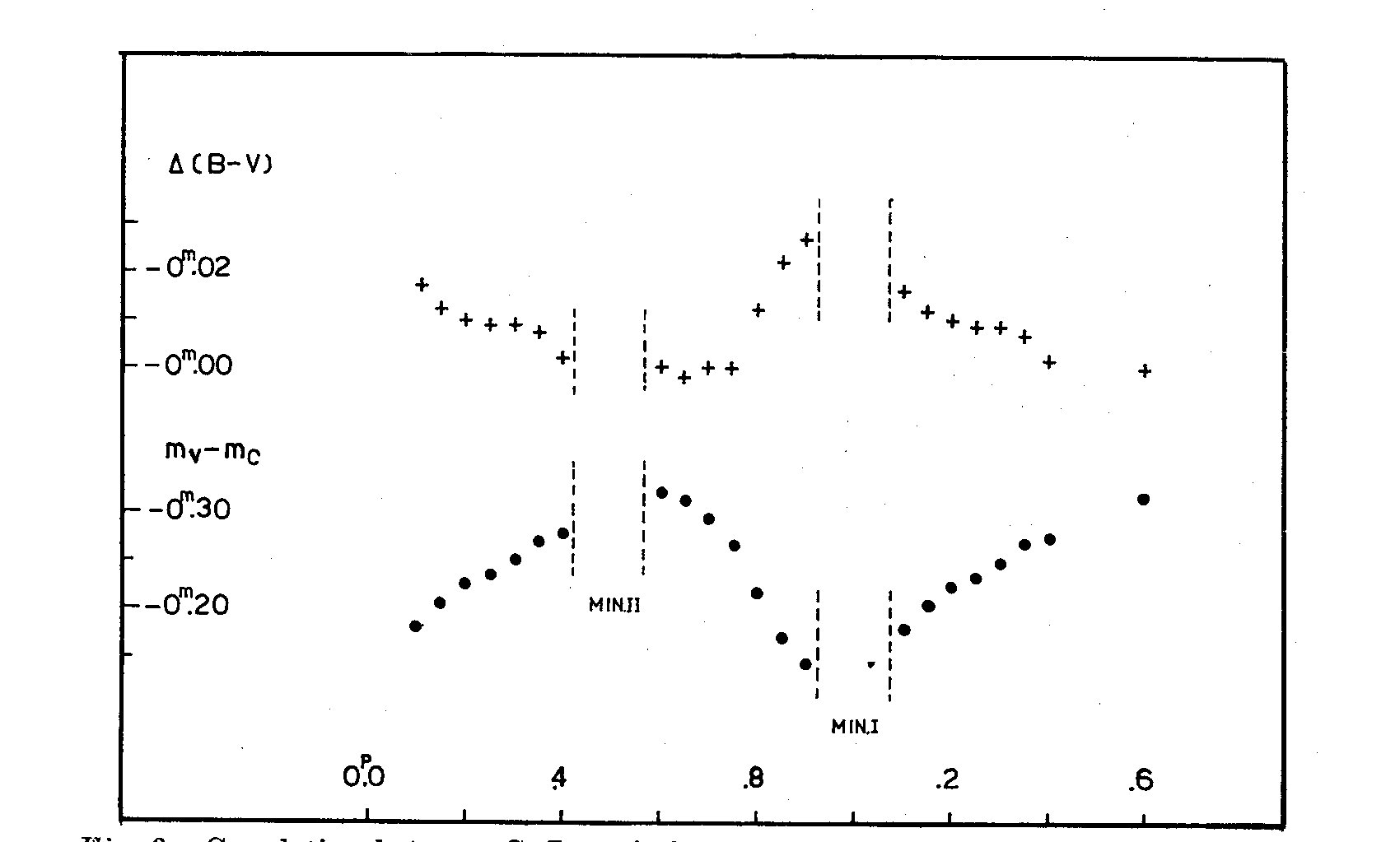 Fig. 3a. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1967 observations).
Fig. 3a. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1967 observations).
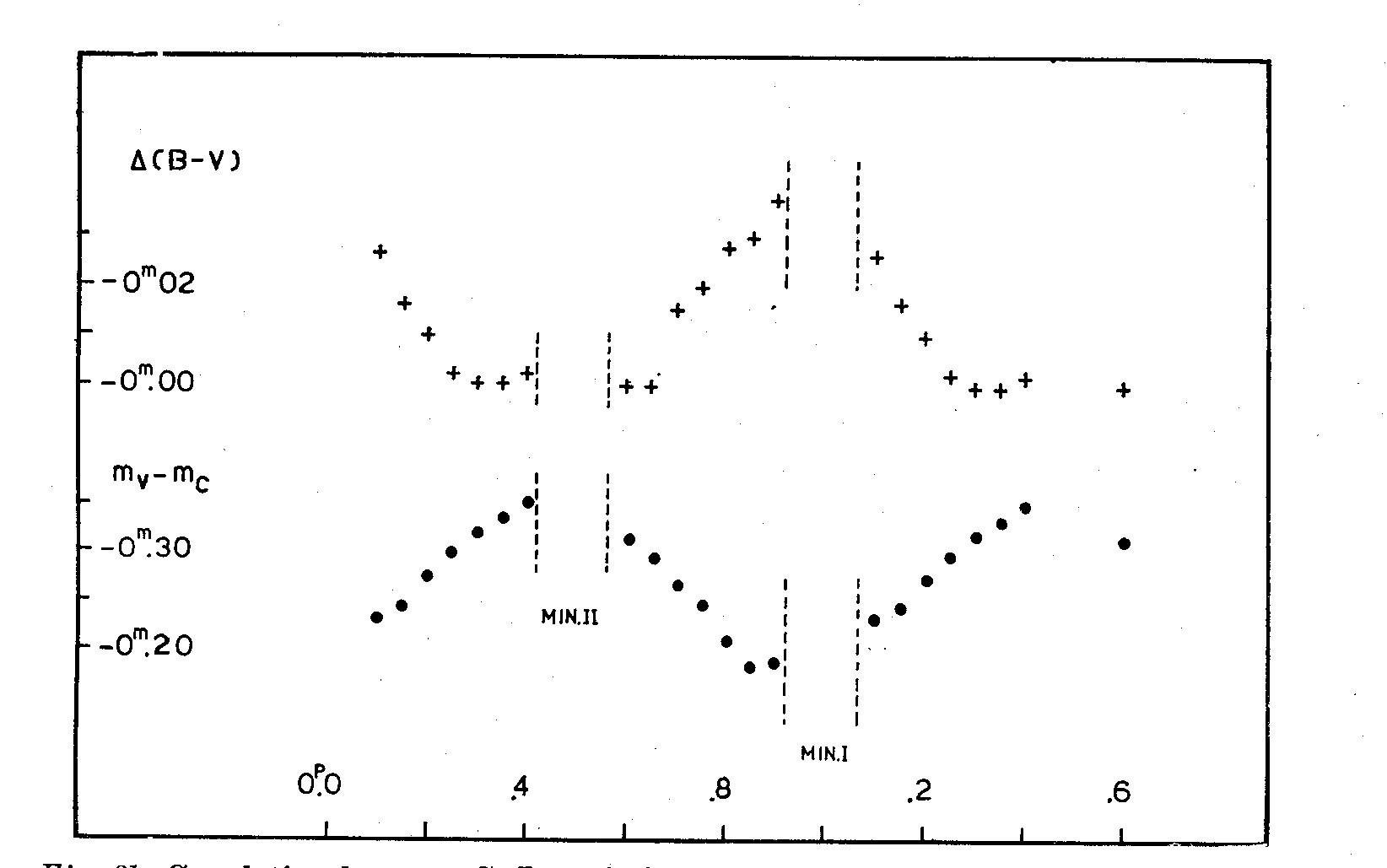 Fig. 3b. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1968 observations).
VARIATION OF DEPTH OF PRIMARY MINIMUM
Many observational evidences give the suspicion that the distortion of
the light curve is due to the secondary component. Fluctuations of the
depth of the primary minimum were already observed by Keller and Limber
and by Popper. These fluctuations are confirmed by our observations. The
primary eclipse is total, therefore these variations are due to the
secondary component.
Fig. 3b. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1968 observations).
VARIATION OF DEPTH OF PRIMARY MINIMUM
Many observational evidences give the suspicion that the distortion of
the light curve is due to the secondary component. Fluctuations of the
depth of the primary minimum were already observed by Keller and Limber
and by Popper. These fluctuations are confirmed by our observations. The
primary eclipse is total, therefore these variations are due to the
secondary component.
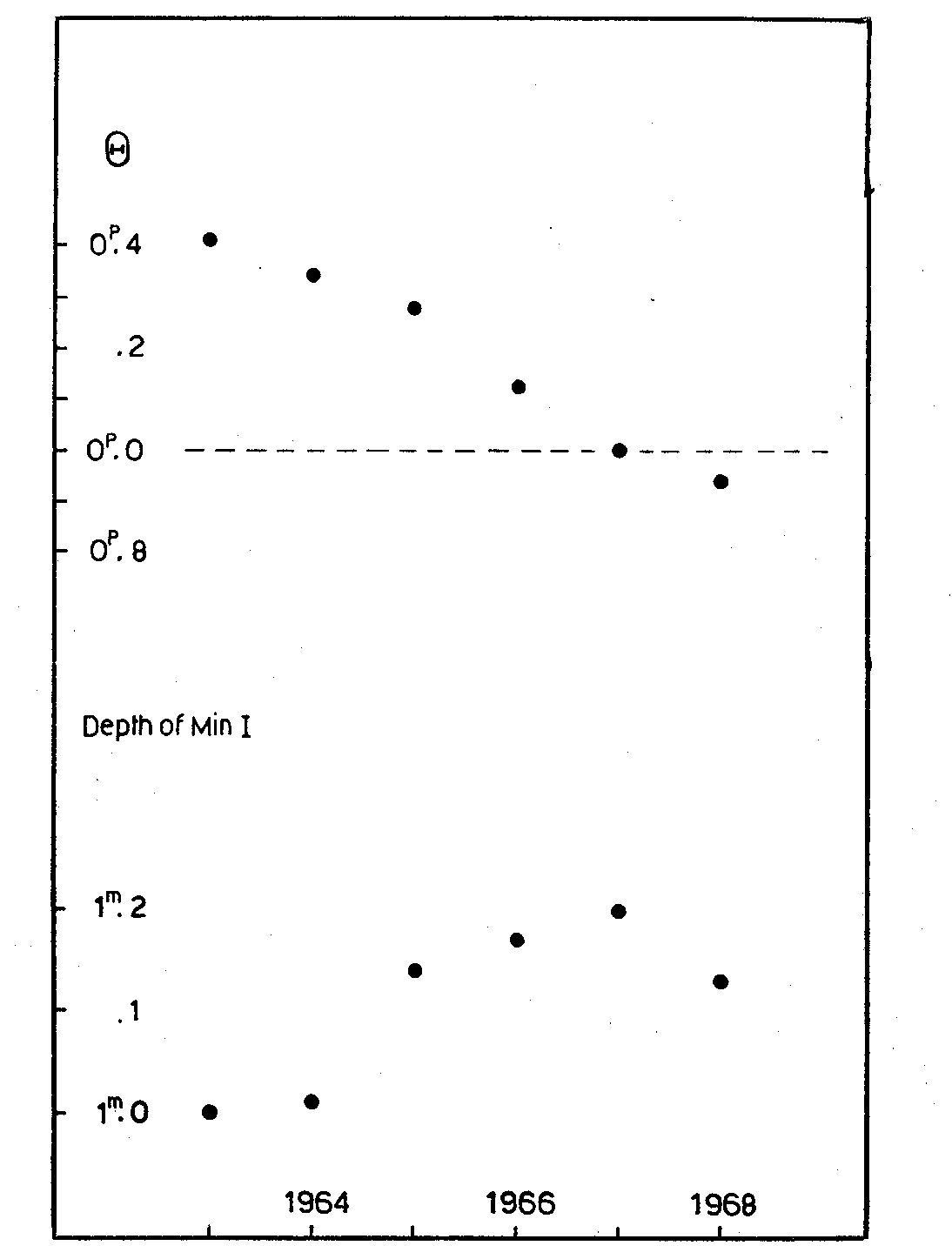 Fig. 4. Correlation between position angle of distortion and depth
of primary minimum.
In Fig. 4 (above) the phases O of minima of the light curve distortion
and (below) the depths of the primary minima are plotted versus time. It
is evident that the variation of the depth of primary minimum is clearly
connected with the position of the distortion in the light curve. In
particular, when the minimum of the distortion falls near the primary
eclipse (i.e., the secondary component is fainter at this phase) the
eclipse appears deeper.
The fluctuation in the luminosity of the secondary component could be
connected in some way with the orbital motion.
ORBITAL PERIOD VARIATION AND DISPLACEMENT OF THE SECONDARY
MINIMUM
From Fig. 5 we can deduce that the fluctuations of the orbital period
around the mean value 4.797865d have a cycle of about 4000 orbital periods
which is little shorter than Plavec' value (Plavec 1960).
Plavec (1960) pointed out that the observed period variation of RS CVn
could. not arise from rotation of one or both components around axes
inclined to the orbital plane.
The third body hypothesis was excluded by Payne-Gaposchkin (1930) and
decisively by Plavec (1960).
It is questionable to attribute the period variation to an eccentricity
of the orbit. In fact the secondary minimum is strongly asymmetrical and this
does not permit an accurate determination of its position, which seems to be
affected by the relative position of the light curve distortion. At present
the period of the displacement of the secondary minimum resulting from our
observations is incompatibly smaller than the orbital period variation.
Fig. 4. Correlation between position angle of distortion and depth
of primary minimum.
In Fig. 4 (above) the phases O of minima of the light curve distortion
and (below) the depths of the primary minima are plotted versus time. It
is evident that the variation of the depth of primary minimum is clearly
connected with the position of the distortion in the light curve. In
particular, when the minimum of the distortion falls near the primary
eclipse (i.e., the secondary component is fainter at this phase) the
eclipse appears deeper.
The fluctuation in the luminosity of the secondary component could be
connected in some way with the orbital motion.
ORBITAL PERIOD VARIATION AND DISPLACEMENT OF THE SECONDARY
MINIMUM
From Fig. 5 we can deduce that the fluctuations of the orbital period
around the mean value 4.797865d have a cycle of about 4000 orbital periods
which is little shorter than Plavec' value (Plavec 1960).
Plavec (1960) pointed out that the observed period variation of RS CVn
could. not arise from rotation of one or both components around axes
inclined to the orbital plane.
The third body hypothesis was excluded by Payne-Gaposchkin (1930) and
decisively by Plavec (1960).
It is questionable to attribute the period variation to an eccentricity
of the orbit. In fact the secondary minimum is strongly asymmetrical and this
does not permit an accurate determination of its position, which seems to be
affected by the relative position of the light curve distortion. At present
the period of the displacement of the secondary minimum resulting from our
observations is incompatibly smaller than the orbital period variation.
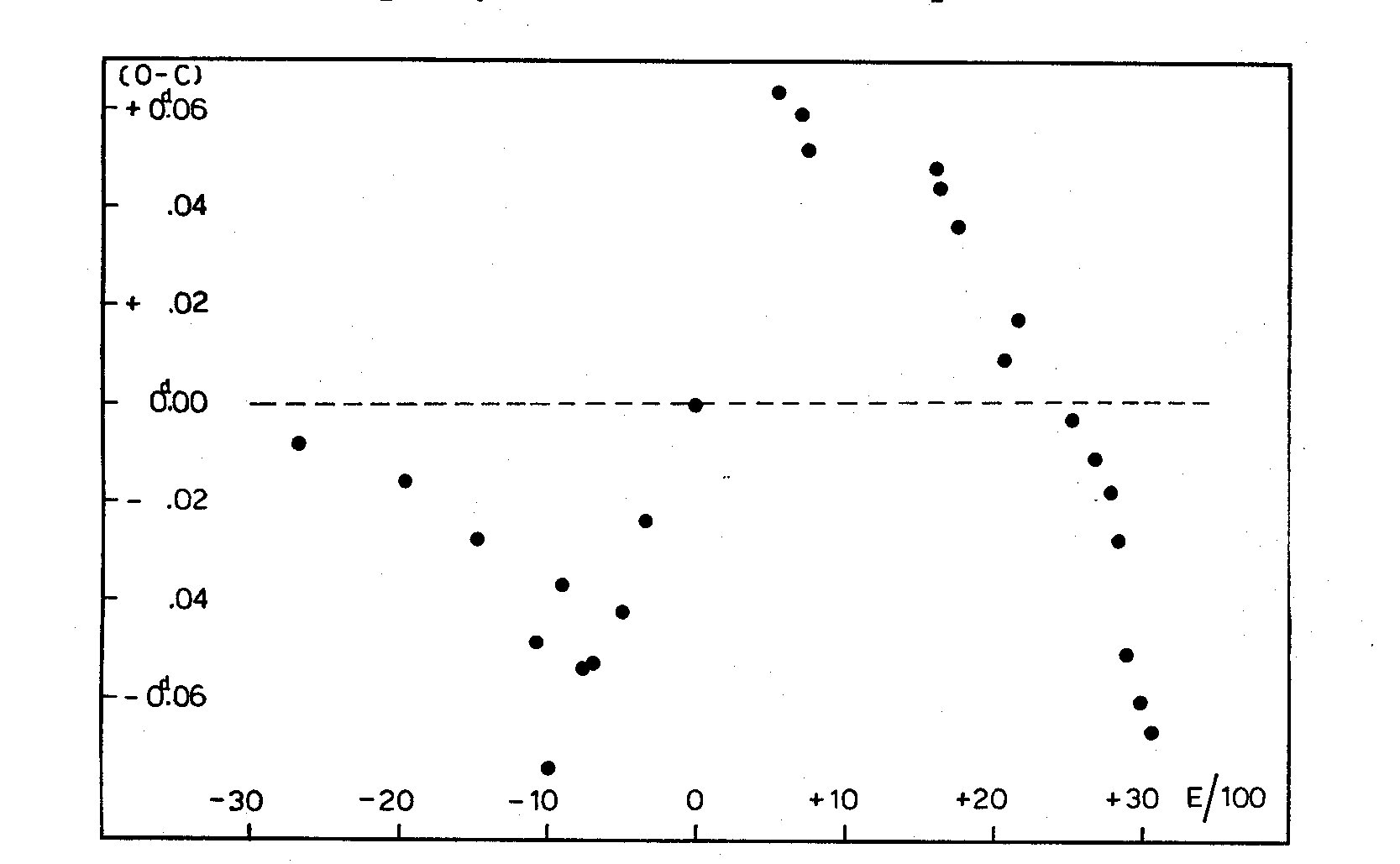 Fig. 5. Period variation.
The radial velocity curves do not show evidence of orbital eccentricity.
Ejection of matter from one or both components seems to be the only
mechanism that may explain the orbital period variation.
SPECTRAL PECULIARITIES
Probably variable emission lines, as H_alpha, H and K lines of ionized
calcium, have been observed in the spectrum of the secondary component
(Hiltner 1947; Joy 1940; Popper 1961).
Azimov (1965) found that the electron density and the temperature of
the secondary component are higher than those of single subgiants of the
same spectral type.
Finally, our unpublished spectroscopic observations carried out at the
Asiago Astrophysical Observatory confirm the presence of the mentioned
emission lines, which do not disappear during the secondary eclipse, as
previously reported by Hiltner (1947).
REFERENCES
Azimov, S. M., 1965, Abastumansk, astrofiz. Obs. Bjull., 33, 81.
Catalano, S., Rodono, M., 1967, Mem. Soc. astr. Ital., 38, 395.
Chisari, D., Lacona, M., 1965, Mem. Soc, astr. ital., 36, 463.
Hiltner, W. A., 1947, Astrophys. J., 106, 481.
Joy, A. A., 1930, Astrophys. J., 72, 41.
Keller, G., Limber, D. N., 1951, Astrophys. J., 113, 637.
Mergentaler, J., 1950, Wroclaw Contr. No. 4.
Payne-Gaposchkin, C., 1939, Harvard Repr. No. 170.
Plavec, M., 1960, Bull. astr. Inst. Csl., 11, 152.
Popper, D. M., 1961, Astrophys. J., 133, 148.
Schneller, H., 1928, Astr. Nachr., 233, 361.
Sitterly, B. W., 1930, Princ. Contr., 11, 21.
Fig. 5. Period variation.
The radial velocity curves do not show evidence of orbital eccentricity.
Ejection of matter from one or both components seems to be the only
mechanism that may explain the orbital period variation.
SPECTRAL PECULIARITIES
Probably variable emission lines, as H_alpha, H and K lines of ionized
calcium, have been observed in the spectrum of the secondary component
(Hiltner 1947; Joy 1940; Popper 1961).
Azimov (1965) found that the electron density and the temperature of
the secondary component are higher than those of single subgiants of the
same spectral type.
Finally, our unpublished spectroscopic observations carried out at the
Asiago Astrophysical Observatory confirm the presence of the mentioned
emission lines, which do not disappear during the secondary eclipse, as
previously reported by Hiltner (1947).
REFERENCES
Azimov, S. M., 1965, Abastumansk, astrofiz. Obs. Bjull., 33, 81.
Catalano, S., Rodono, M., 1967, Mem. Soc. astr. Ital., 38, 395.
Chisari, D., Lacona, M., 1965, Mem. Soc, astr. ital., 36, 463.
Hiltner, W. A., 1947, Astrophys. J., 106, 481.
Joy, A. A., 1930, Astrophys. J., 72, 41.
Keller, G., Limber, D. N., 1951, Astrophys. J., 113, 637.
Mergentaler, J., 1950, Wroclaw Contr. No. 4.
Payne-Gaposchkin, C., 1939, Harvard Repr. No. 170.
Plavec, M., 1960, Bull. astr. Inst. Csl., 11, 152.
Popper, D. M., 1961, Astrophys. J., 133, 148.
Schneller, H., 1928, Astr. Nachr., 233, 361.
Sitterly, B. W., 1930, Princ. Contr., 11, 21.
 Fig. 1. Observed light curves.
Fig. 1. Observed light curves.
 Fig. 2. Position angle of light curve distortion.
Fig. 2 shows the Theta values versus the number of orbital period from an
assumed initial epoch (JD 2425249.028; Schneller, 1928). Because of the
uncertainty of the first three values of Theta it is difficult to determine
the exact period in which Theta reaches the same value. On the hypotheses
that Keller and Limber's observations and ours up to 1966 belong to the
same cycle, we made a rough estimate of 2400 orbital periods (Catalano
and Rodono 1967). Using new observations obtained during 1967 and 1968,
it seems that Keller and Limber's observations belong to a preceding
cycle. Therefore a more suitable value might be about 800 orbital
periods. Limiting ourselves to the Catania observations this period
would be even smaller.
COLOUR INDEX OUTSIDE ECLIPSES
The amplitude of the distortion is larger at longer wavelengths. The
data in the following Table refer to the observations of 1968:
U B V
0.10m 0.14m 0.17m
Consequently the light outside eclipses is bluer at its minimum than at
its maximum. This appears clearly in Figs. 3a and 3b, where for 1967 and
1968 the mean light curves and the colour index variations Delta(B -V), both
outside eclipses, are reported.
This behaviour of the colour index, as observed in many systems showing
distortions of their light curves, has been discussed by Mergentaler (1950).
He supposed that gaseous streams of negative ions of hydrogen of different
optical thickness could cause the observed distortions in the light curve.
If the distortion of RS CVn is due to this reason, the absorbing matter should
have an equilibrium configuration, because the wave-like distortion, as
we have seen, maintains its shape with time. We had suggested (Catalano
and Rodono 1967) that a ring around the equatorial plane of the primary
component might cause the distortion of the light curve and its shift
with time. This idea was supported primarily by the good agreement
between the previously determined period of the distortion shift (2400 P)
and the theoretical period of precession of the equator, which was
assumed to have inclined to the orbital plane. But the new estimate of
the period of the light variation, as previously reported, is too short
to be compared with the theoretical one.
Fig. 2. Position angle of light curve distortion.
Fig. 2 shows the Theta values versus the number of orbital period from an
assumed initial epoch (JD 2425249.028; Schneller, 1928). Because of the
uncertainty of the first three values of Theta it is difficult to determine
the exact period in which Theta reaches the same value. On the hypotheses
that Keller and Limber's observations and ours up to 1966 belong to the
same cycle, we made a rough estimate of 2400 orbital periods (Catalano
and Rodono 1967). Using new observations obtained during 1967 and 1968,
it seems that Keller and Limber's observations belong to a preceding
cycle. Therefore a more suitable value might be about 800 orbital
periods. Limiting ourselves to the Catania observations this period
would be even smaller.
COLOUR INDEX OUTSIDE ECLIPSES
The amplitude of the distortion is larger at longer wavelengths. The
data in the following Table refer to the observations of 1968:
U B V
0.10m 0.14m 0.17m
Consequently the light outside eclipses is bluer at its minimum than at
its maximum. This appears clearly in Figs. 3a and 3b, where for 1967 and
1968 the mean light curves and the colour index variations Delta(B -V), both
outside eclipses, are reported.
This behaviour of the colour index, as observed in many systems showing
distortions of their light curves, has been discussed by Mergentaler (1950).
He supposed that gaseous streams of negative ions of hydrogen of different
optical thickness could cause the observed distortions in the light curve.
If the distortion of RS CVn is due to this reason, the absorbing matter should
have an equilibrium configuration, because the wave-like distortion, as
we have seen, maintains its shape with time. We had suggested (Catalano
and Rodono 1967) that a ring around the equatorial plane of the primary
component might cause the distortion of the light curve and its shift
with time. This idea was supported primarily by the good agreement
between the previously determined period of the distortion shift (2400 P)
and the theoretical period of precession of the equator, which was
assumed to have inclined to the orbital plane. But the new estimate of
the period of the light variation, as previously reported, is too short
to be compared with the theoretical one.
 Fig. 3a. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1967 observations).
Fig. 3a. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1967 observations).
 Fig. 3b. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1968 observations).
VARIATION OF DEPTH OF PRIMARY MINIMUM
Many observational evidences give the suspicion that the distortion of
the light curve is due to the secondary component. Fluctuations of the
depth of the primary minimum were already observed by Keller and Limber
and by Popper. These fluctuations are confirmed by our observations. The
primary eclipse is total, therefore these variations are due to the
secondary component.
Fig. 3b. Correlation between C. I. variation (+) and the mean V light curve
outside eclipses (filled circle) (1968 observations).
VARIATION OF DEPTH OF PRIMARY MINIMUM
Many observational evidences give the suspicion that the distortion of
the light curve is due to the secondary component. Fluctuations of the
depth of the primary minimum were already observed by Keller and Limber
and by Popper. These fluctuations are confirmed by our observations. The
primary eclipse is total, therefore these variations are due to the
secondary component.
 Fig. 4. Correlation between position angle of distortion and depth
of primary minimum.
In Fig. 4 (above) the phases O of minima of the light curve distortion
and (below) the depths of the primary minima are plotted versus time. It
is evident that the variation of the depth of primary minimum is clearly
connected with the position of the distortion in the light curve. In
particular, when the minimum of the distortion falls near the primary
eclipse (i.e., the secondary component is fainter at this phase) the
eclipse appears deeper.
The fluctuation in the luminosity of the secondary component could be
connected in some way with the orbital motion.
ORBITAL PERIOD VARIATION AND DISPLACEMENT OF THE SECONDARY
MINIMUM
From Fig. 5 we can deduce that the fluctuations of the orbital period
around the mean value 4.797865d have a cycle of about 4000 orbital periods
which is little shorter than Plavec' value (Plavec 1960).
Plavec (1960) pointed out that the observed period variation of RS CVn
could. not arise from rotation of one or both components around axes
inclined to the orbital plane.
The third body hypothesis was excluded by Payne-Gaposchkin (1930) and
decisively by Plavec (1960).
It is questionable to attribute the period variation to an eccentricity
of the orbit. In fact the secondary minimum is strongly asymmetrical and this
does not permit an accurate determination of its position, which seems to be
affected by the relative position of the light curve distortion. At present
the period of the displacement of the secondary minimum resulting from our
observations is incompatibly smaller than the orbital period variation.
Fig. 4. Correlation between position angle of distortion and depth
of primary minimum.
In Fig. 4 (above) the phases O of minima of the light curve distortion
and (below) the depths of the primary minima are plotted versus time. It
is evident that the variation of the depth of primary minimum is clearly
connected with the position of the distortion in the light curve. In
particular, when the minimum of the distortion falls near the primary
eclipse (i.e., the secondary component is fainter at this phase) the
eclipse appears deeper.
The fluctuation in the luminosity of the secondary component could be
connected in some way with the orbital motion.
ORBITAL PERIOD VARIATION AND DISPLACEMENT OF THE SECONDARY
MINIMUM
From Fig. 5 we can deduce that the fluctuations of the orbital period
around the mean value 4.797865d have a cycle of about 4000 orbital periods
which is little shorter than Plavec' value (Plavec 1960).
Plavec (1960) pointed out that the observed period variation of RS CVn
could. not arise from rotation of one or both components around axes
inclined to the orbital plane.
The third body hypothesis was excluded by Payne-Gaposchkin (1930) and
decisively by Plavec (1960).
It is questionable to attribute the period variation to an eccentricity
of the orbit. In fact the secondary minimum is strongly asymmetrical and this
does not permit an accurate determination of its position, which seems to be
affected by the relative position of the light curve distortion. At present
the period of the displacement of the secondary minimum resulting from our
observations is incompatibly smaller than the orbital period variation.
 Fig. 5. Period variation.
The radial velocity curves do not show evidence of orbital eccentricity.
Ejection of matter from one or both components seems to be the only
mechanism that may explain the orbital period variation.
SPECTRAL PECULIARITIES
Probably variable emission lines, as H_alpha, H and K lines of ionized
calcium, have been observed in the spectrum of the secondary component
(Hiltner 1947; Joy 1940; Popper 1961).
Azimov (1965) found that the electron density and the temperature of
the secondary component are higher than those of single subgiants of the
same spectral type.
Finally, our unpublished spectroscopic observations carried out at the
Asiago Astrophysical Observatory confirm the presence of the mentioned
emission lines, which do not disappear during the secondary eclipse, as
previously reported by Hiltner (1947).
REFERENCES
Azimov, S. M., 1965, Abastumansk, astrofiz. Obs. Bjull., 33, 81.
Catalano, S., Rodono, M., 1967, Mem. Soc. astr. Ital., 38, 395.
Chisari, D., Lacona, M., 1965, Mem. Soc, astr. ital., 36, 463.
Hiltner, W. A., 1947, Astrophys. J., 106, 481.
Joy, A. A., 1930, Astrophys. J., 72, 41.
Keller, G., Limber, D. N., 1951, Astrophys. J., 113, 637.
Mergentaler, J., 1950, Wroclaw Contr. No. 4.
Payne-Gaposchkin, C., 1939, Harvard Repr. No. 170.
Plavec, M., 1960, Bull. astr. Inst. Csl., 11, 152.
Popper, D. M., 1961, Astrophys. J., 133, 148.
Schneller, H., 1928, Astr. Nachr., 233, 361.
Sitterly, B. W., 1930, Princ. Contr., 11, 21.
Fig. 5. Period variation.
The radial velocity curves do not show evidence of orbital eccentricity.
Ejection of matter from one or both components seems to be the only
mechanism that may explain the orbital period variation.
SPECTRAL PECULIARITIES
Probably variable emission lines, as H_alpha, H and K lines of ionized
calcium, have been observed in the spectrum of the secondary component
(Hiltner 1947; Joy 1940; Popper 1961).
Azimov (1965) found that the electron density and the temperature of
the secondary component are higher than those of single subgiants of the
same spectral type.
Finally, our unpublished spectroscopic observations carried out at the
Asiago Astrophysical Observatory confirm the presence of the mentioned
emission lines, which do not disappear during the secondary eclipse, as
previously reported by Hiltner (1947).
REFERENCES
Azimov, S. M., 1965, Abastumansk, astrofiz. Obs. Bjull., 33, 81.
Catalano, S., Rodono, M., 1967, Mem. Soc. astr. Ital., 38, 395.
Chisari, D., Lacona, M., 1965, Mem. Soc, astr. ital., 36, 463.
Hiltner, W. A., 1947, Astrophys. J., 106, 481.
Joy, A. A., 1930, Astrophys. J., 72, 41.
Keller, G., Limber, D. N., 1951, Astrophys. J., 113, 637.
Mergentaler, J., 1950, Wroclaw Contr. No. 4.
Payne-Gaposchkin, C., 1939, Harvard Repr. No. 170.
Plavec, M., 1960, Bull. astr. Inst. Csl., 11, 152.
Popper, D. M., 1961, Astrophys. J., 133, 148.
Schneller, H., 1928, Astr. Nachr., 233, 361.
Sitterly, B. W., 1930, Princ. Contr., 11, 21.